Have you ever noticed when Hollywood seems to produce two similar movies at the same time? Armageddon and Deep Impact. Mission to Mars and Red Planet. Tombstone and Wyatt Earp. Maybe this is great minds thinking alike. Maybe it's a sign that Hollywood is unimaginative.
Anyway, a couple of weeks ago, I mentioned that Veritasium had made a video about the trickiest problem in probability. Now Numberphile tackles the same problem and comes to the same solution. Great minds etc.
I've laid out my thoughts on this in another post and am not going to retread the same ground. So, here's a little twist to further illustrate the result.
Remember that the problem is that Sleeping Beauty is recruited for an experiment. On Sunday, she is put to sleep and a fair coin is tossed. If the coin comes up heads, she is woken up on Monday and put back to sleep. If it's tails, she is woken up on Monday and Tuesday. On Wednesday, she is awoken and the experiment is concluded.
When she is awoken during the experiment, she is asked one question before her memory is blanked and she is put back to sleep. That question is "What is the probability that the result of the coin toss was heads?" You can read about the discussion over at wikipedia.
As I noted previously, it is important to realise that the important thing is the question - Given that you are awake, what was the probability that the coin toss came up heads?
Let's generalize the problem to take place over n days. If the coin comes up heads, Sleeping Beauty is woken just once. If it is tails, then she is woken n times.
The calculations are quite straightforward and I'll leave that as an exercise for the reader, but the result is that the probability that the coin came up heads given that Sleeping Beauty is asked is:

and clearly, the probability that the coin came up tails is:
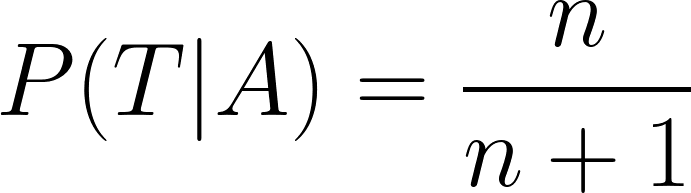
When n=2, which is the original Sleeping Beauty problem, these two probabilities are 1/3 and 2/3 respectively.
But let's think a little about what happens if n is large, say a thousand days. If you were Sleeping Beauty and were told you would be woken a thousand times if the coin came up tails, but only once it were a heads, then if you are asked then clearly it is far more likely that you would say it was a tails.
This trickiest problem doesn't seem particularly tricky.